(2sinx*cosx)/((cosx)^2 (sinx)^2) then wherever i go with it, it leadsSimplify\\frac {\sec (x)\sin^2 (x)} {1\sec (x)} simplify\\sin^2 (x)\cos^2 (x)\sin^2 (x) simplify\\tan^4 (x)2\tan^2 (x)1 simplify\\tan^2 (x)\cos^2 (x)\cot^2 (x)\sin^2 (x)If you're doing this by de Moivre, the trick is to keep the form you get from initially expanding (CiS)^3, (where C = cos x, S = sin x) rather than rewriting to get sin 3x in terms of only sin x ie (CiS)^3 = C^3 3i C^2S 3 C S^2 iS^3 So sin 3x =3 C^2 S S^3, cos 3x = C^33CS^2 And Then just divide by C^3 to rewrite in terms of tan x
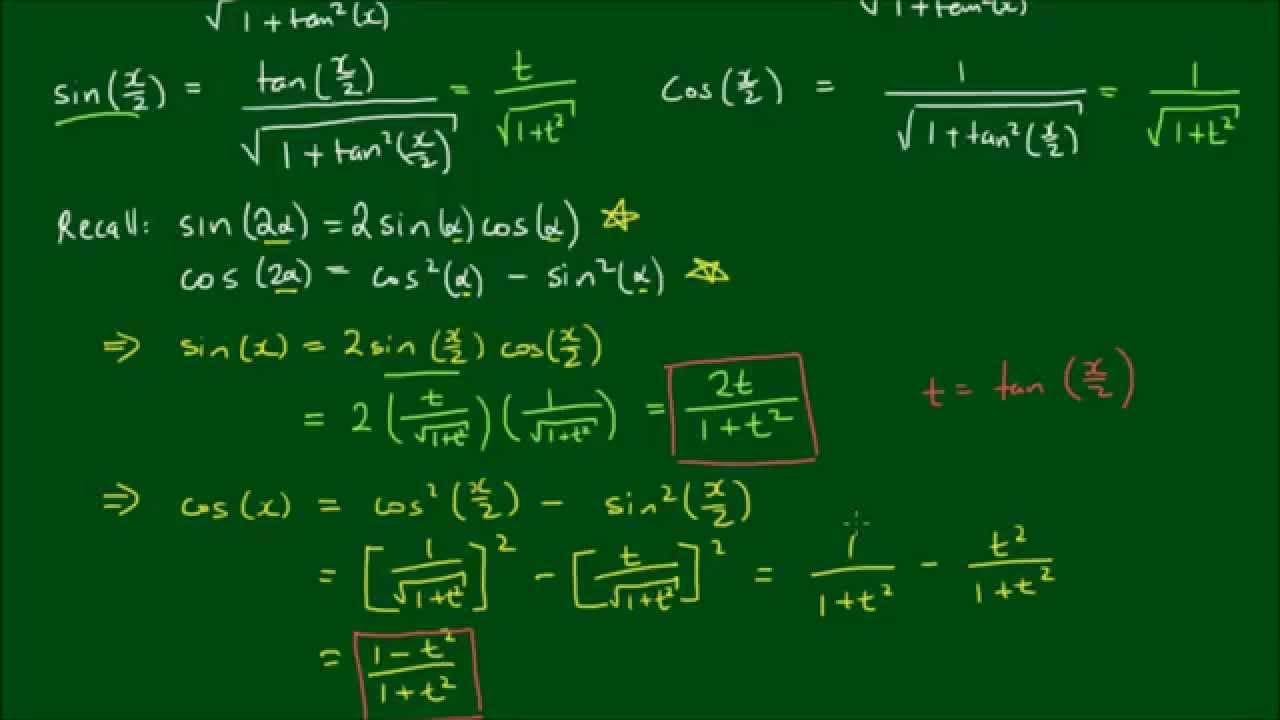
Expressing Sin X And Cos X In Terms Of T Tan X 2 Youtube
Tan 2x formula in terms of cos x
Tan 2x formula in terms of cos x-Integral of cos^2x We can't just integrate cos^2(x) as it is, so we want to change it into another form, which we can easily do using trig identities Integral of cos^2(2x) Recall the double angle formula cos(2x) = cos^2(x) – sin^2(x) We also know the trig identity sin^2(x) cos^2(x) = 1, so combining these we get the equationY 1 cos2x 2 cos2x sin x dx y sin5x cos2x dx y sin2x 2 cos2x sin x dx halfangle formula for , however, we have Notice that we mentally made the substitution when integrating Another terms of tangent using the identity We can then evaluate the integral by substituting with 1 7 tan 7x 1 9 tan 9x C u7 7 u9 9 C



1
Free trigonometric equation calculator solve trigonometric equations stepbystepTan2x Formulas Tan2x Formula = 2 tan x 1 − t a n 2 x We know that tan (x) = sin (x)/cos (x)Formulas from Trigonometry sin 2Acos A= 1 sin(A B) = sinAcosB cosAsinB cos(A B) = cosAcosB tansinAsinB tan(A B) = A tanB 1 tanAtanB sin2A= 2sinAcosA cos2A= cos2 A sin2 A tan2A= 2tanA 1 2tan A sin A 2 = q 1 cosA 2 cos A 2
• take the Pythagorean equation in this form, sin2 x = 1 – cos2 x and substitute into the First doubleangle identity cos 2x = cos2 x – sin2 x cos 2x = cos2 x – (1 – cos2 x) cos 2x = cos 2 x – 1 cos 2 x cos 2x = 2cos 2 x – 1 Third doubleangle identity for cosine Summary of DoubleAngles • Sine sin 2x = 2 sin xThe trigonometric formulas like Sin2x, Cos 2x, Tan 2x are popular as double angle formulae, because they have double angles in their trigonometric functions For solving many problems we may use these widely The Sin 2x formula is \(Sin 2x = 2 sin x cos x\) Where x is the angle Source enwikipediaorg Derivation of the FormulaThe cosine of double angle is equal to the quotient of the subtraction of square of tangent from one by the sum of one and square of tan function cos 2 θ = 1 − tan 2 θ 1 tan 2 θ It is called the cosine of double angle identity in terms of tangent function
The formula given in my book does not seem to work in Mathcad Prime 30 In the book there is no multiplier (*) printed after tan^2 and cos^2 There is just empty space I did change the formula around in all kinds of ways I put tan inside parenthesis like (tan)^2, or (tan^2* (gammaQ)), or (tan (gammaQ)^2) but nothing works Indicated Solution We can derive the Weierstrass Substitution Using the tangent double angle formula $$ \tan(x)=\frac{2t}{1t^2}\tag{1} $$ Then writing $\sec^2(xTan (2x) is a doubleangle trigonometric identity which takes the form of the ratio of sin (2x) to cos (2x) sin (2x) = 2 sin (x) cos (x) cos (2x) = (cos (x))^2 – (sin (x))^2 = 1 – 2 (sin (x))^2 = 2 (cos (x))^2 – 1 Proof 71K views · View upvotes · View shares




Tan2x Sin2x Tan2x Sin2x 5 1 Brainly In




Rewrite The Expression In Terms Of The First Power Of The Cosine Cos 4xtan 2x Brainly Com
Proportionality constants are written within the image sin θ, cos θ, tan θ, where θ is the common measure of five acute angles In mathematics, the trigonometric functions (also called circular functions, angle functions or goniometric functions) are real functions which relate an angle of a rightangled triangle to ratios of two side lengths hmm, sin/cos=tan, cos/sin=cot, sin^2 cos^2=1 i need cos(theta) in terms of tan(theta) though Unless thats what we are working up to )I wanted to find $\tan2x$ in terms of $\cos x$ alone I was able to do it in terms of $\sin x$ alone $\tan2x = \sin2x/\cos2x$ Since, $\cos2x = 12\sin^2x$ Therefore, $\tan2x = (\sin2x / 12\sin^2x)$ Is it possible to do it in terms of $\cos x$ alone ?



Trig Find Tan X Given Tan 2x 2 Pi X 3pi 2 Cheatatmathhomework




X 0tan2x Sin2x X 3 Maths Questions
Let cosx = Gand cos x lies below the x axis Find sin(2x),cos(2x) and tan(2x) b Proof the formula of unit circler y2 = 1, and explain it in your own wards (6 marks) (12 marks) 2 2 A boy is flying two kites at the same time He has (250m) feet of line out to one kite and (3m) feet to the otherX in Quadrant I sin 2x cos 2x = XAnswer and Explanation 1 Given 1−cos2x cosx⋅tanx 1 − cos 2 x cos x ⋅ tan x Simplifying the above expression as below




Calculate Sin2x Cos2x And Tan2x For Given Tanx In Quadrant 2 Youtube




Ex 3 4 8 Find General Solution Of Sec 2 2x 1 Tan 2x Teachoo
Trigonometric substitutions are a specific type of u u u substitutions and rely heavily upon techniques developed for those They use the key relations sin 2 x cos 2 x = 1 \sin^2x \cos^2x = 1 sin2 xcos2 x = 1, tan 2 x 1 = sec 2 x \tan^2x 1 = \sec^2x tan2 x 1 = sec2 x, and cot 2 x 1 = csc 2 xIn #29 & 30, find sin 2x, cos 2x, tan x = cosx = — and tan 2x using the given information 30 sec x = 2;Tan(3x) in terms of tan(x), write tan(3x) in terms of tan(x), using the angle sum formula and the double angle formulas, simplifying trig identities, trigono




Use Identities To Rewrite Cosx Sinxtanx In Terms Of Sin X And Cos X Then Simplify So That No Quotients Appear Wyzant Ask An Expert



2
Double Angle Formulas The trigonometric double angle formulas give a relationship between the basic trigonometric functions applied to twice an angle in terms of trigonometric functions of the angle itself Tips for remembering the following formulas We can substitute the values ( 2 x) (2x) (2x) into the sum formulas for sin \sin sin andCos(x)^2(1tan(x)^2)=1 Replace the with based on the identity Simplify each term Pull terms out from under the radical, assuming positive real numbers The period of the function can be calculated using Replace with in the formula for period Solve the equationCos 2x = (1tan^2 x)/(1 tan^2 x)` Plugging `tan x = sqrt6/3` in the formulas above yields
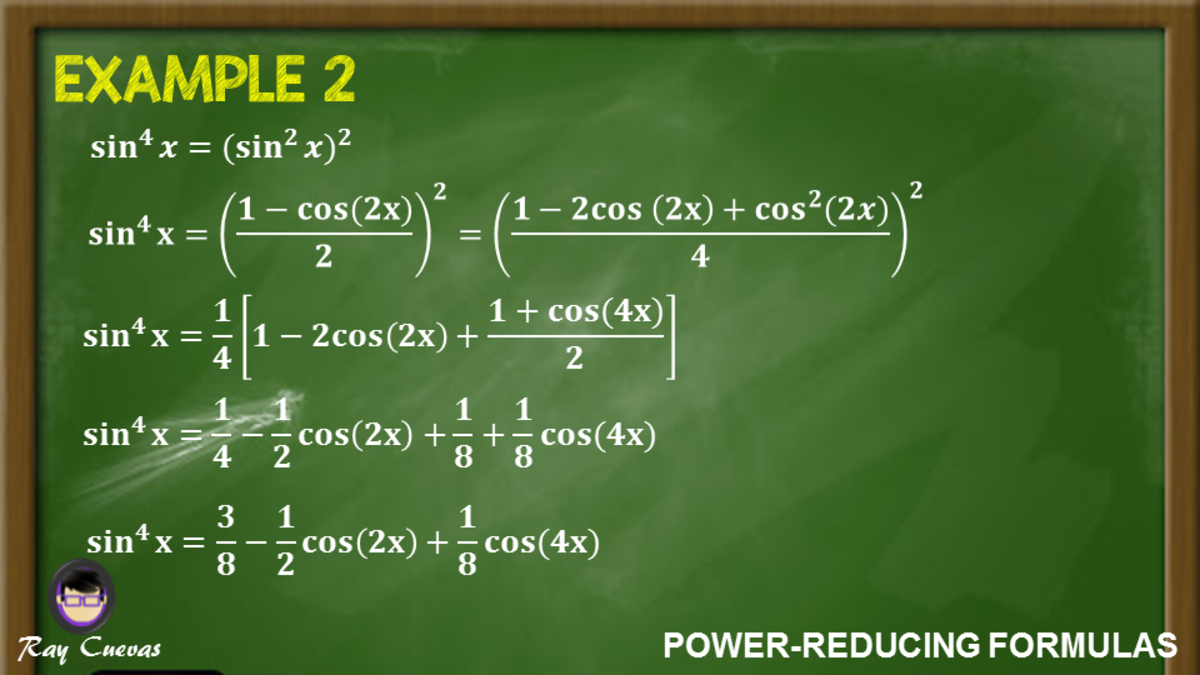



Power Reducing Formulas And How To Use Them With Examples Owlcation



Http Theprofessorshut Weebly Com Uploads 2 3 2 7 Pc Chap 6 Sect 2 Sum Difference And Cofunction Identities Pdf
Sin x < 0 29 2SthktOSL Cos2x x in Q2 sin 2x = cos2x = tan 2x = — 1(5E IS sin 2x = cos 2x = 25 tan 2x = sin— = 10 cos— = 10 31 Find tan 2x if cscx = 4 and tan x < 0 In #32 & 33, find sin£ and cosE using the given informationYou need to write sin 2x and cos 2x in terms of tanx such that `sin 2x = (2 tan x)/(1 tan^2 x); The most straightforward way to obtain the expression for cos (2 x) is by using the "cosine of the sum" formula cos (x y) = cosx*cosy sinx*siny To get cos (2 x), write 2x = x x



Solved A First Rewrite The Left Hand Side 1 Tan 2x In Terms Of Sin X And Cos X B Next Add The Two Terms In The Expression You Got In Part
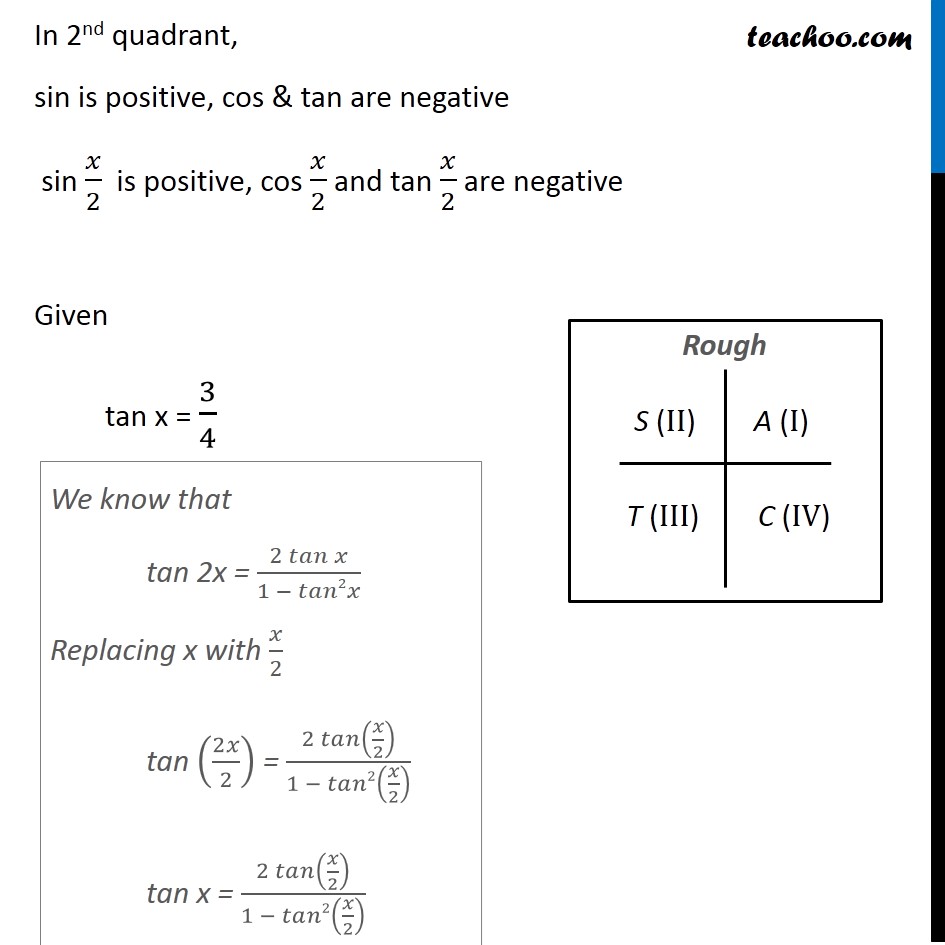



Example 28 If Tan X 3 4 Find Sin X 2 Cos X 2 Tan X 2
The double angle formulas can be derived by setting A = B in the sum formulas above For example, sin(2A) = sin(A)cos(A) cos(A)sin(A) = 2sin(A)cos(A) It is common to see two other forms expressing cos(2A) in terms of the sine and cosine of the single angle A Recall the square identity sin 2 (x) cos 2 (x) = 1 from Sections 14 and 23Solve for x tan(2x)=(sin(2x))/(cos(2x)) Divide each term in the equation by Rewrite in terms of sines and cosines Rewrite as a product Multiply and Simplify the denominator The tangent function is positive in the first and third quadrantsTan2x Formula Sin 2x, Cos 2x, Tan 2x is the trigonometric formulas which are called as double angle formulas because they have double angles in their trigonometric functions Let's understand it by practicing it through solved example




Answered For The Following Exercises Find The Bartleby



1
Answer Formulas that express the trigonometric functions of an angle 2x in terms of functions of an angle x at trigonometric formulae are known as the double angle formulae They are called 'double angle' because they consist of trigonometric functions ofSin (θ), Tan (θ), and 1 are the heights to the line starting from the x axis, while Cos (θ), 1, and Cot (θ) are lengths along the x axis starting from the origin The functions sine, cosine and tangent of an angle are sometimes referred to as the primary or basic trigonometric functions Explanation using the trigonometric identities ∙ xtanθ = sinθ cosθ ∙ xsin2θ cos2θ = 1 ⇒ sinθ = ± √1 − cos2θ tanθ = sinθ cosθ = ± √1 −cos2θ cosθ Answer link



Www Sbac Edu Cms Lib Fl Centricity Domain 1591 Pdf



Francisjosephcampena Weebly Com Uploads 1 7 8 6 Ched Precalculus Part5 Pdf
Using following trigonometric identities Sinx^2Cosx^2==1 Sinx/Cosx==Tanx Cscx==1/Si Stack Exchange Network Stack Exchange network consists of 178 Q&A communities including Stack Overflow , the largest, most trusted online community for developers to learn, share their knowledge, and build their careersA particle P moves on the xaxis At t seconds, its velocity is v = t^2 7t 10 a) At what times is P at rest, b) what is P's maximum speed in the interval 0≤ t≤ 4, and c) what is the total distance P has moved between t=0 and t=4The range of cscx is the same as that of secx, for the same reasons (except that now we are dealing with the multiplicative inverse of sine of x, not cosine of x)Therefore the range of cscx is cscx ‚ 1 or cscx • ¡1 The period of cscx is the same as that of sinx, which is 2Since sinx is an odd function, cscx is also an odd function Finally, at all of the points where cscx is
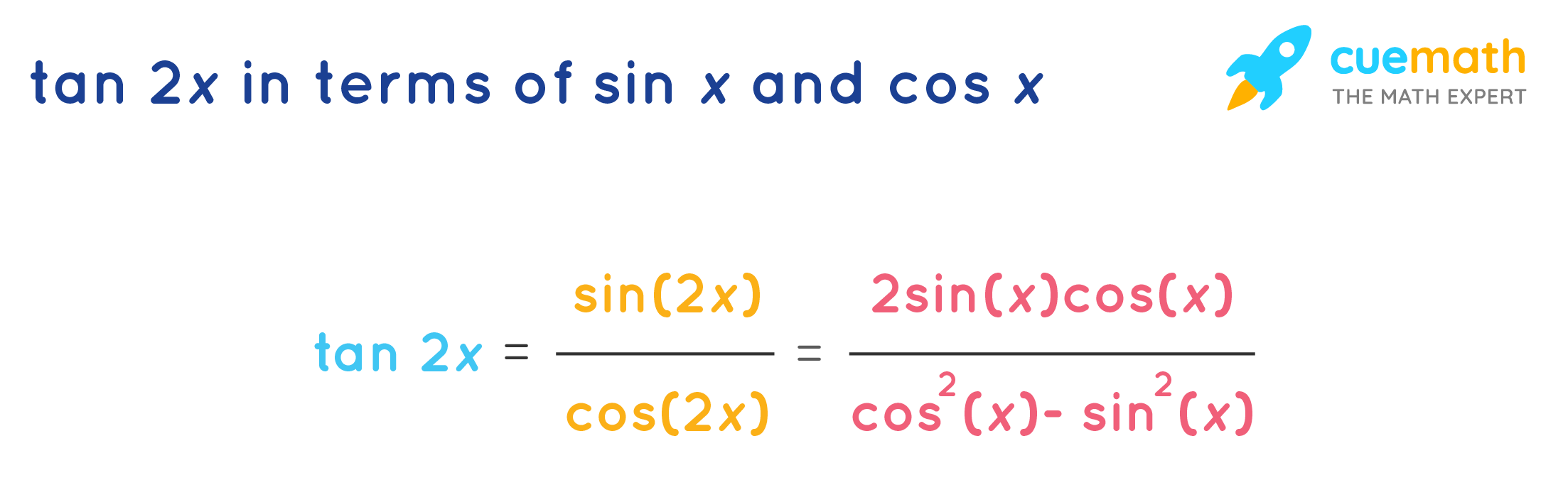



Tan 2x Formula What Is Tan 2x Formula Examples




Derivative Rules For Trigonometric Functions
Question Use the formulas for lowering powers to rewrite the expression in terms of the first power of cosine, cos4 x sin2 x Find sin 2x, cos 2x, and tan 2x from the given information COS X = 15 17 11 CSC X < 0 sin 2x = COS 2X= tan 2x = Find sin 2x, cos 2x, and tan 2x from the given informatic sin x = 8 17!Cos x = (e ix eix)/2 sin x = (e ix eix)/2i cosh x = (e x ex)/2 sinh x = (e x ex)/2 tan x = sin x / cos x tanh x = sinh x / cosh x cot x = cos x / sin x coth x = cosh x / sinh x cos 2 x sin 2 x = 1 cosh 2 x sinh 2 x = 1 d(e x)/dx = e x d(cos x)/dx = sin x d(sin x)/dx = cos x d(cosh x)/dx = sinh x d(sinh x)/dx = cosh x e ix = cos x i sin x cos ix = cosh x\(\cos 2X = \cos ^{2}X – \sin ^{2}X \) Hence, the first cos 2X formula follows, as \(\cos 2X = \cos ^{2}X – \sin ^{2}X\) And for this reason, we know this formula as double the angle formula, because we are doubling the angle Other Formulae of cos 2X \(\cos 2X = 1 – 2 \sin ^{2}X \) To derive this, we need to start from the earlier derivation




How Do You Prove The Identity Tan 2x Secx 1 1 Cosx Cosx Socratic



2
In Trigonometry Formulas, we will learn Basic Formulas sin, cos tan at 0, 30, 45, 60 degrees Pythagorean Identities Sign of sin, cos, tan in different quandrants Radians Negative angles (EvenOdd Identities) Value of sin, cos, tan repeats after 2π Shifting angle by π/2, π, 3π/2 (CoFunction Identities or Periodicity Identities)Formula sin 2 θ = 2 tan θ 1 tan 2 θ A trigonometric identity that expresses the expansion of sine of double angle function in terms of tan function is called the sine of double angle identity in tangent functionSin2 (x) = 1 − cos (2x) 2 cos2 (x) = 1 cos (2x) 2 Reduction Formulas ∫ sinn (x)dx = − sinn−1(x) cos (x) n n − 1 n ∫ sinn−2 (x)dx ∫ cosn (x)dx = cosn−1(x) sin (x) 12K views




Expressing Sin X And Cos X In Terms Of T Tan X 2 Youtube




Double Angle Formula Sine Cosine And Tangent Owlcation
Homework Statement Express tan(2x) in terms of sin(x) alone assuming pi < x < 3pi/2 Homework Equations Trig identities The Attempt at a Solution sin2x/cos2x switched for double angle equations;In this video, I show how with a right angled triangle with hypotenuse 1, sides (a) and (b), and using Pythagoras' Theorem, thatcos(x) = 1 / sqrt( 1 tan^2243 The Substitution z = tan (x/2) Suppose our integrand is a rational function of sin (x) and cos (x) After the substitution z = tan (x / 2) we obtain an integrand that is a rational function of z, which can then be evaluated by partial fractions
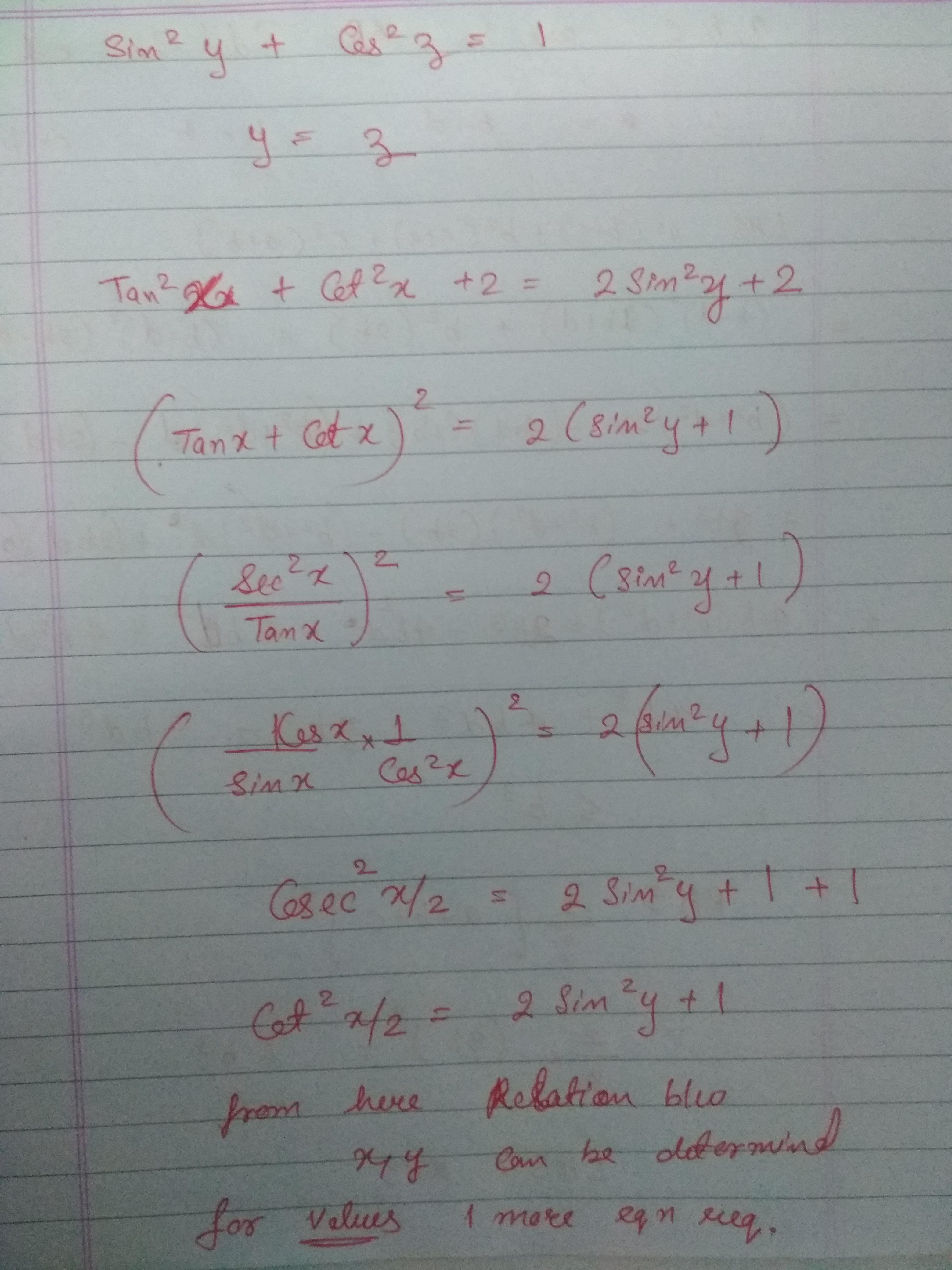



Solve For X Y And Z If Tan 2x Cot 2x 2sin 2y And Sin 2y Cos 2z 1 Askiitians




How Do You Solve 1 Tan 2x 6 2sec 2x Socratic
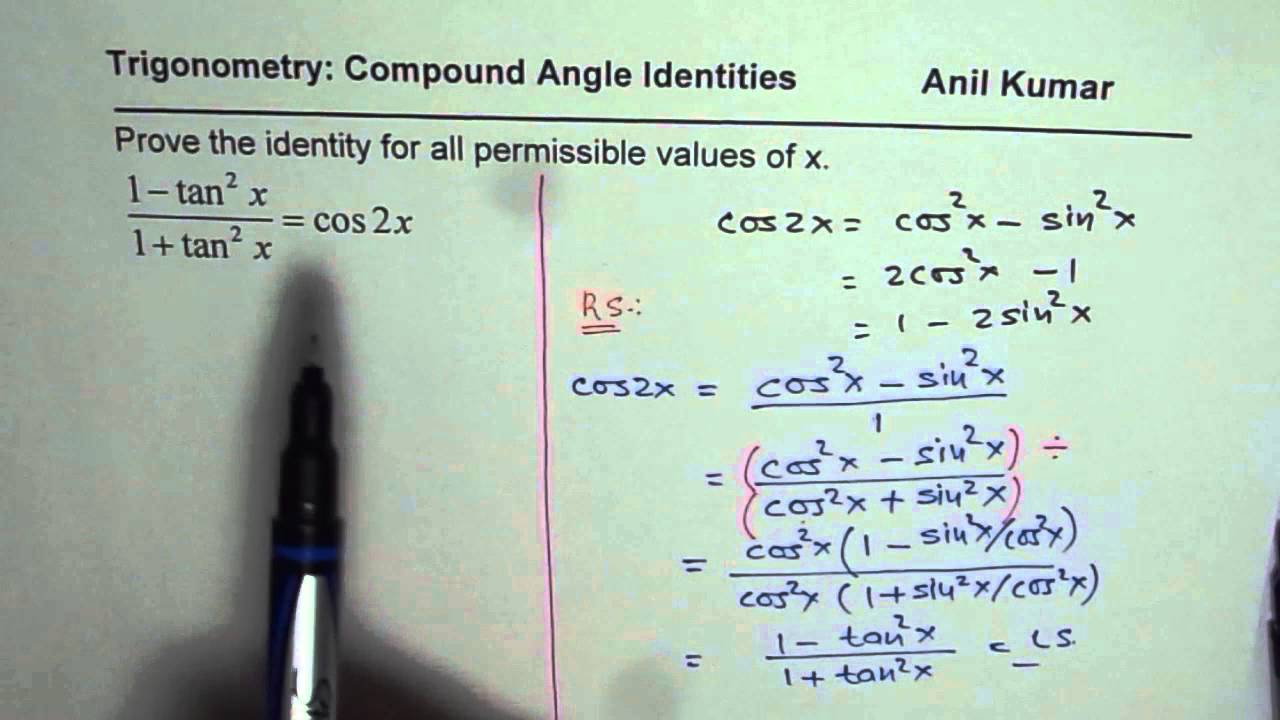



Write Cos2x In Tan And Prove Trigonometric Identity For Double Angle Youtube
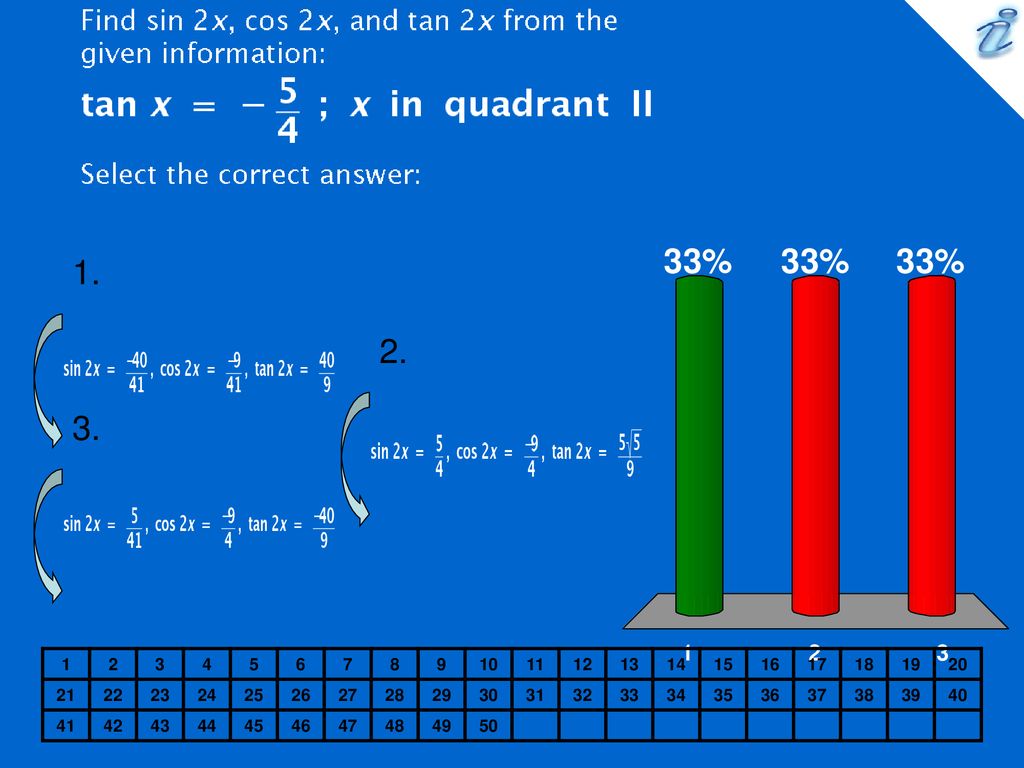



Find Sin 2x Cos 2x And Tan 2x From The Given Information Image Select The Correct Answer Ppt Download




Inttanx Tan2x Tan3x Dx Is Equal To




Tan 2x Formula What Is Tan 2x Formula Examples




Trig Identity Sec2x Minus Tan2x T10 Youtube
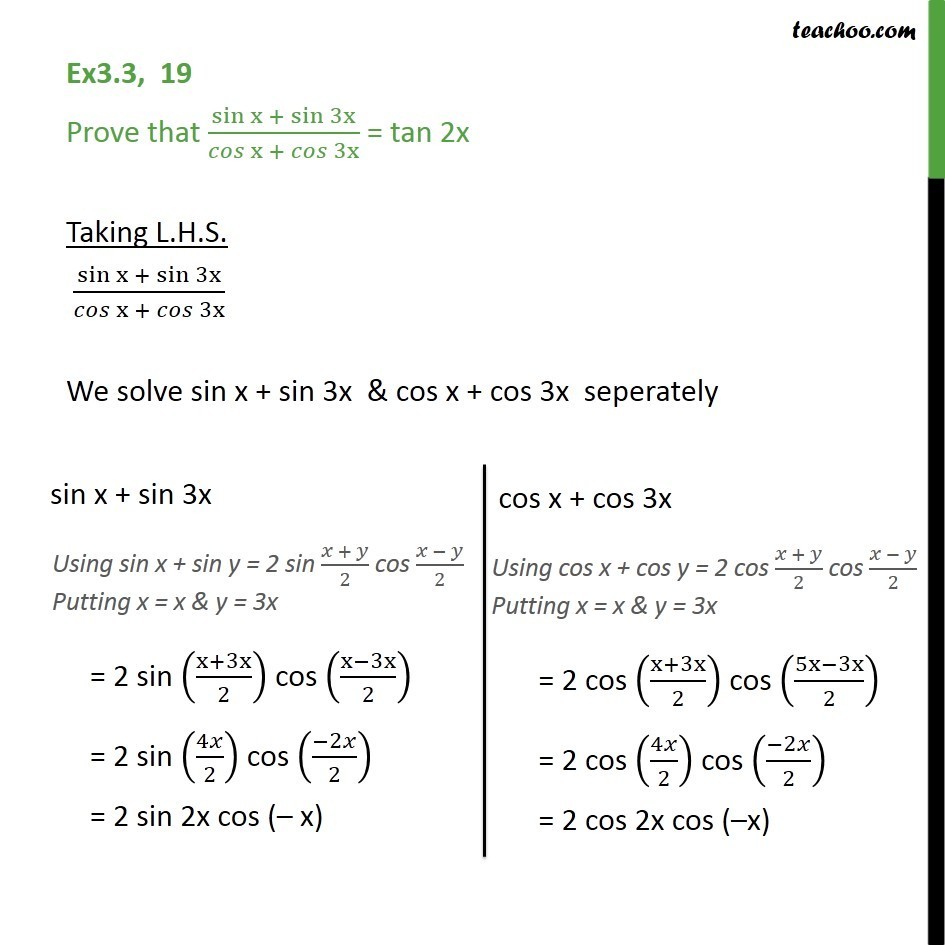



Ex 3 3 19 Prove Sin X Sin 3x Cos X Cos 3x Tan 2x
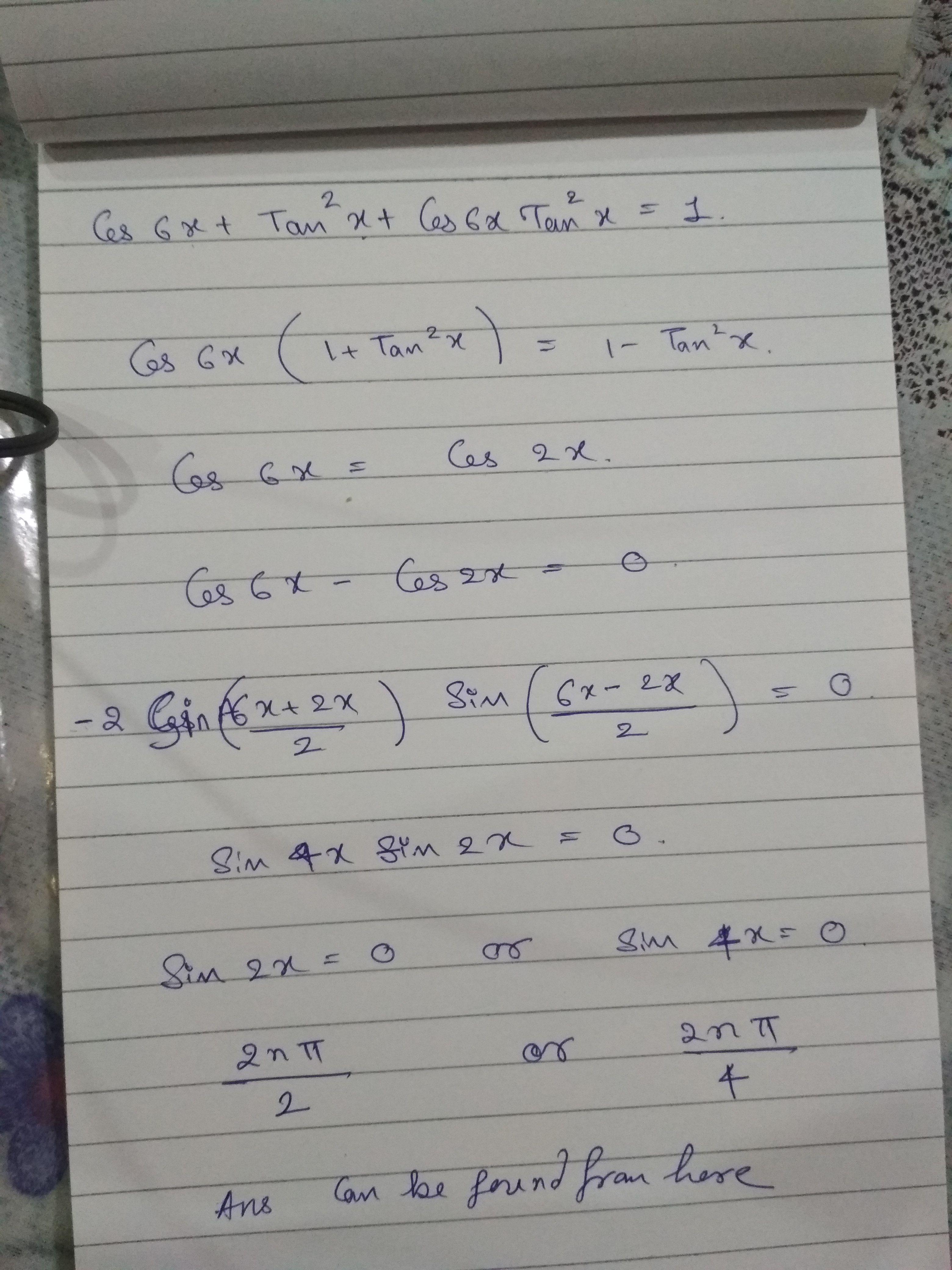



Find The Number Of Solutions Of The Equation Cos 6x Tan 2x Cos 6x Askiitians



Www Math Purdue Edu Kyochman Ma Lesson14 Notes Implicitdifferentiation Pdf



Www Jensenmath Ca Unit 5 pretest review solutions Pdf




How Do You Prove The Identities Cosx Secx Sinx Cscx Sec 2x Tan 2x Socratic




New Syllabus Additional Mathematics Pages 301 350 Flip Pdf Download Fliphtml5




Find The Derivative Of The Given Function Y Tan 2x 1 Cot 2x I Tried Converting The Original Function In Terms Of Sin And Cos But It Was Still Too Complicated To Be Called Simplified




Sin X 2 Tan X 2 1 Tan 2 X 2 Eraoco
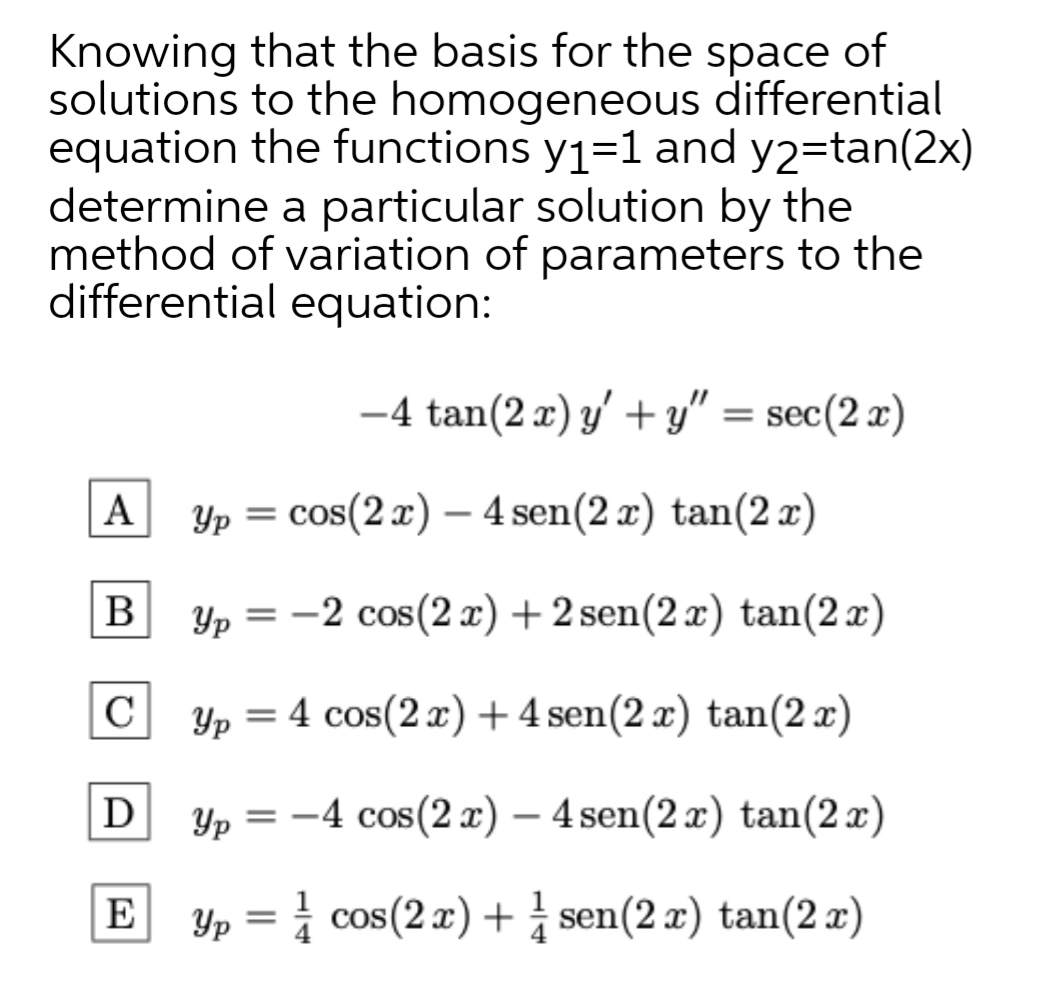



Answered Knowing That The Basis For The Space Of Bartleby



Http Ougouag Com Trigreviewforcalc Pdf




Lesson 4 5 Double Angle Formulas



Www Math Tamu Edu Irinaholmes M133 7p2 Trigintegrals Pdf




Edexcel Core Mathematics C2 June 12 Solutions Examples Worksheets Videos Activities



Q Tbn And9gcrzz4 1ds9ppsd8y 0vi 8exu9yrzt2issbxxz9kcs1yppfojoc Usqp Cau



What Is The Nth Derivative Of Cosx Quora



2



Www Birmingham K12 Mi Us Cms Lib Mi Centricity Domain 976 Chapter 5 Pdf




4 Cos Xcos X Tan X Sin X 5 Tan 2x Tan 2x Sin 2 X 6 Gauthmath



Http Www Sequoiahs Org Documents 1seqfix Ibmathhl Pdf




Answered 1 Find Sin 2x Cos 2x And Tan 2x If Bartleby



Search Q Sin2x In Terms Of Tanx Tbm Isch
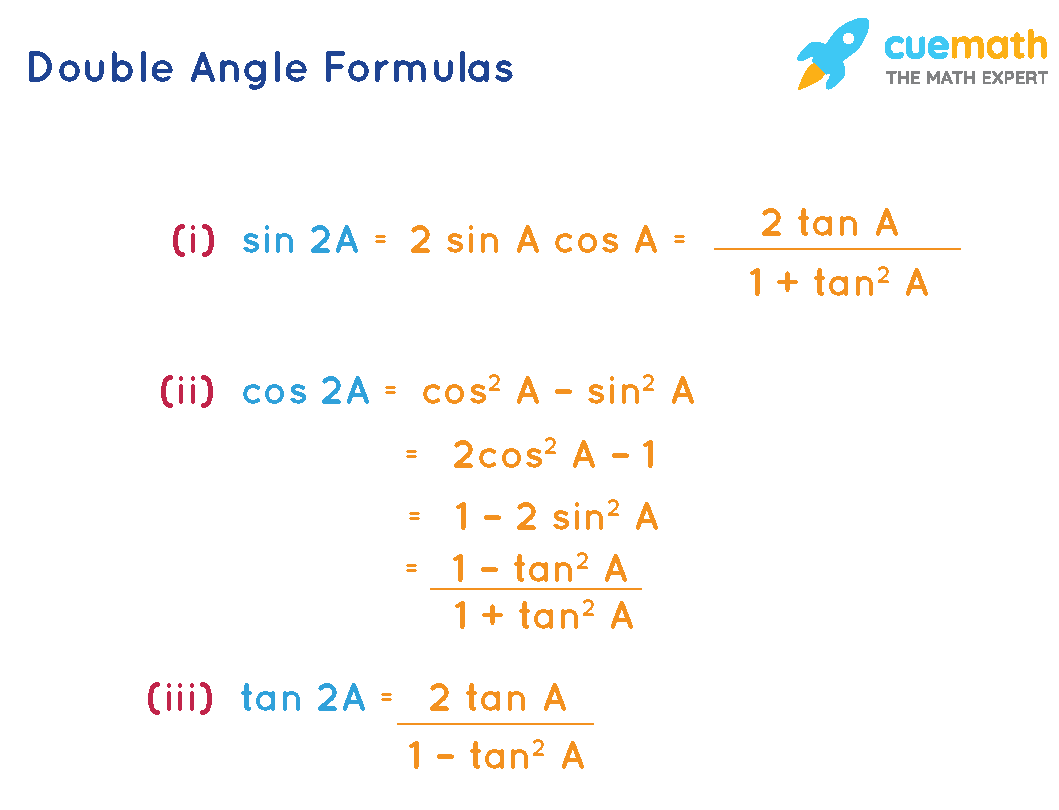



Double Angle Formulas What Are Double Angle Formulas Examples



How To Solve D 2 4 Y Tan2x Quora
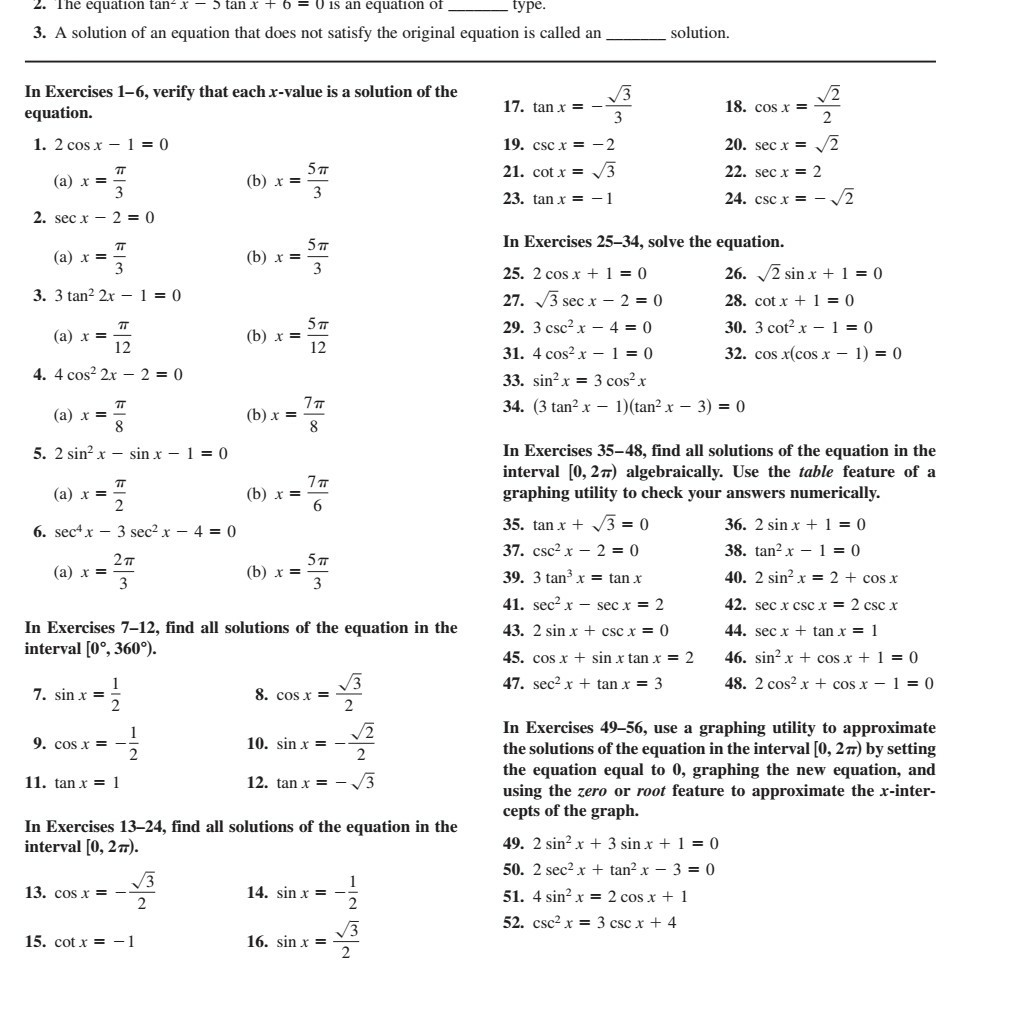



In Exercises 1 6 Verify That Each X Value Is A Chegg Com
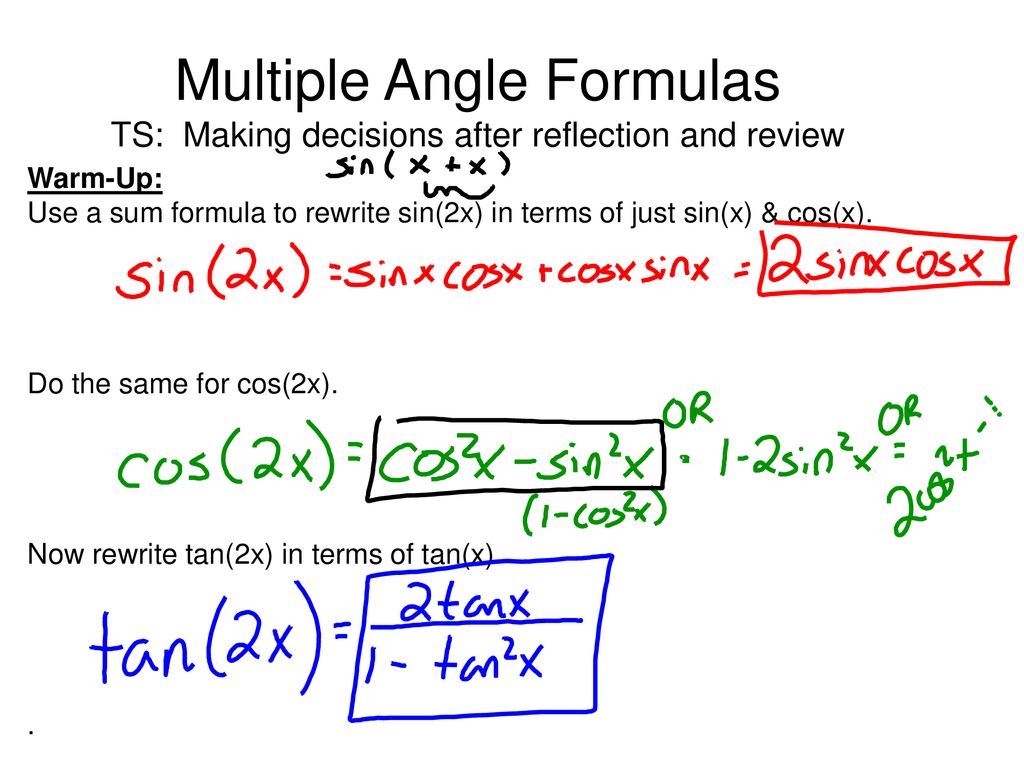



Multiple Angle Formulas Ts Making Decisions After Reflection And Review Warm Up Use A Sum Formula To Rewrite Sin 2x In Terms Of Just Sin X Cos X Ppt Download




If 0 X Pi 2 Then Tan 2x 6 Log Sec X 2 Cos X 4 Is Greater




Ex 3 3 23 Prove Tan 4x 4 Tan X 1 Tan2 X 1 6tan2x




Answered Find Sin 2x Cos 2x And Tan 2x If Sin Bartleby
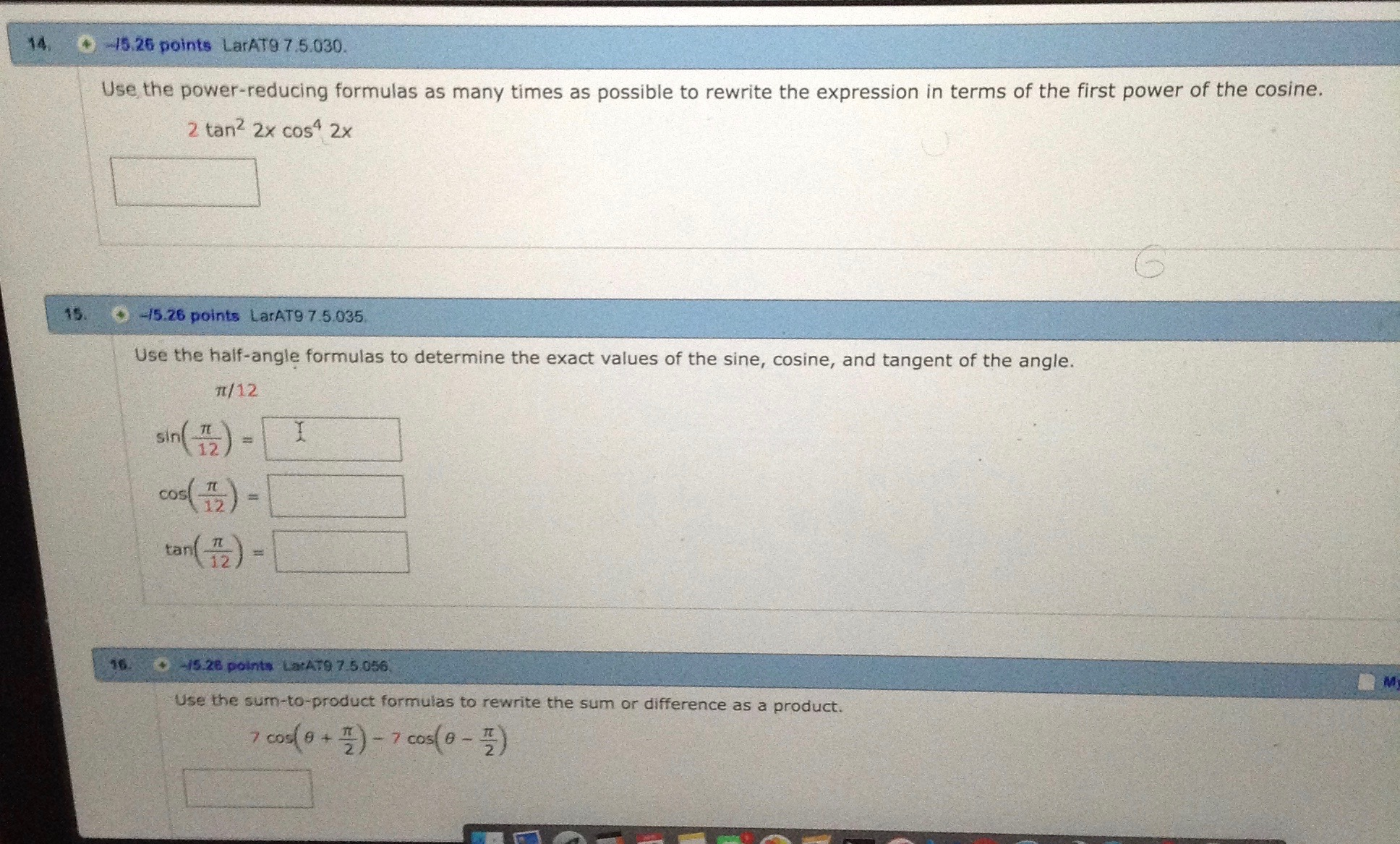



Use The Power Reducing Formulas As Many Times As Chegg Com
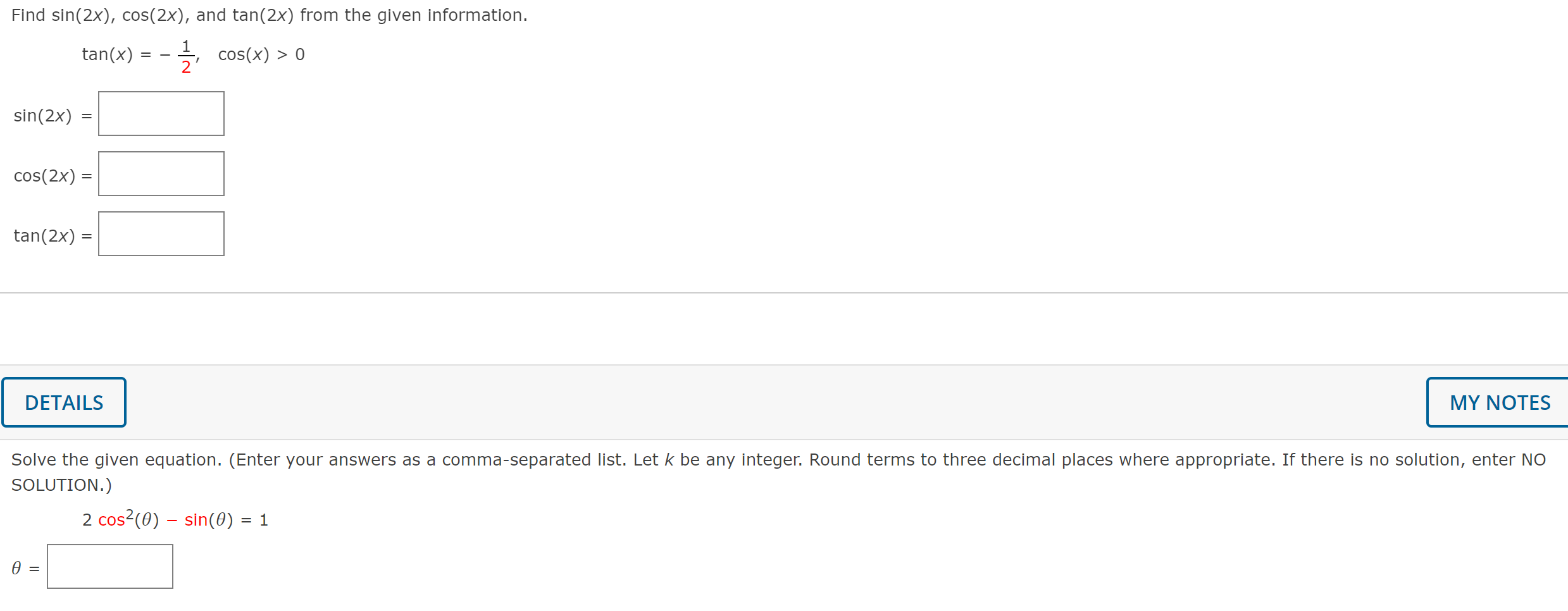



Find Sin 2x Cos 2x And Tan 2x From The Given Chegg Com



Www Thatonemathguy Com Uploads 7 0 1 3 Precalculus 2nd Edition Part 2 Pdf



Http Www Nicolet Us Faculty Dgibbon Identityreviewanswer Pdf




9 3 Other Identities Ppt Download
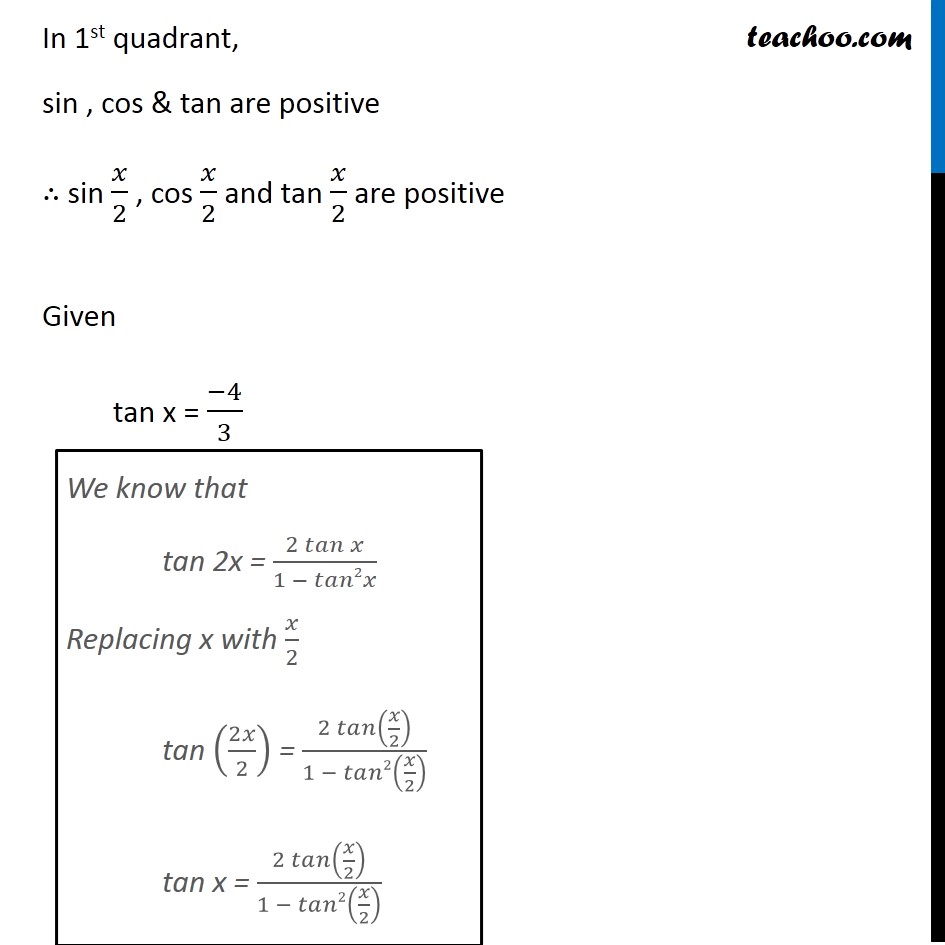



Misc 8 Tan X 4 3 Find Sin X 2 Cos X 2 And Tan X 2




Problem 4 Using The Sin And Cos Addition Formulas Chegg Com




Trigonometry Review Questions Answers Rev 0 Sine Triangle




Solve The Trigonometric Equation Tan X Tan 2x Tan X Tan 2x 1



Tan 2x Tan 2x




Prove That Frac Sec8x 1 Sec4x 1 Frac Tan8x Tan2x Snapsolve
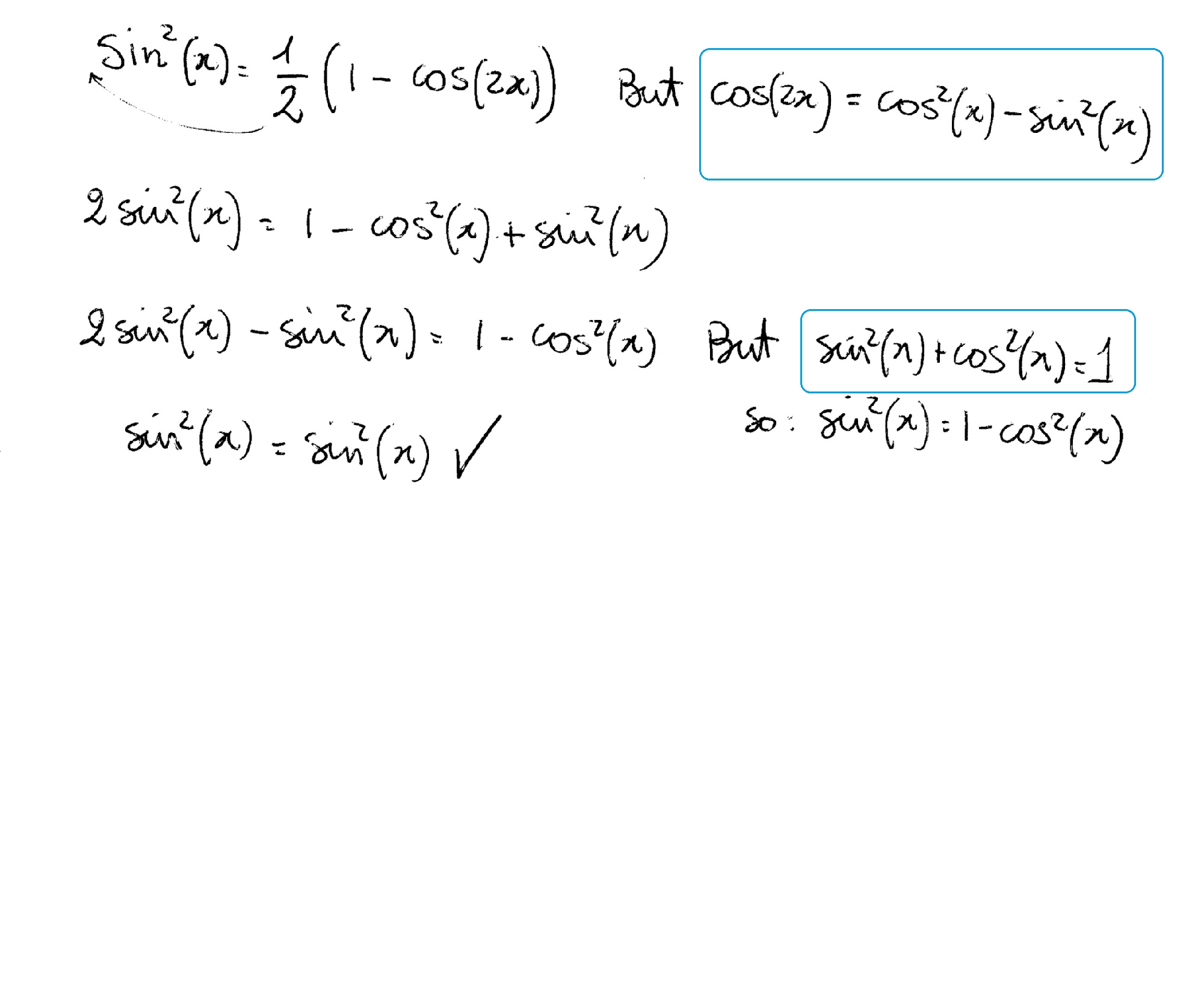



How Do You Verify Sin 2 X 1 2 1 Cos2x Socratic



Www Mulemath Com Pc Book Pg 394 5 Pdf




Powers Of Trigonometric Functions




Dana Harrington Benjamin Leis Thanks So Much For Responding But I Don T Think That S The Case All 6 Solutions Work In The Original Equation When Subbed In Also When You Graph




Trig Identity Tan X 1 Tan 2 X Sec X Cos X Csc X Sin X Proved From Both Sides Youtube



3




Section 7 3 Double Angle Half Angle And Chegg Com




New Syllabus Additional Mathematics Pages 301 350 Flip Pdf Download Fliphtml5



Www Leonschools Net Site Handlers Filedownload Ashx Moduleinstanceid 641 Dataid 1170 Filename Precalc 2 week review packet 3 25 Pdf




Find Sin 2x Cos 2x And Tan 2x If Sinx 5 13 And X Terminates In Quadrant I Ala Sin 2x U H Cos Brainly Com
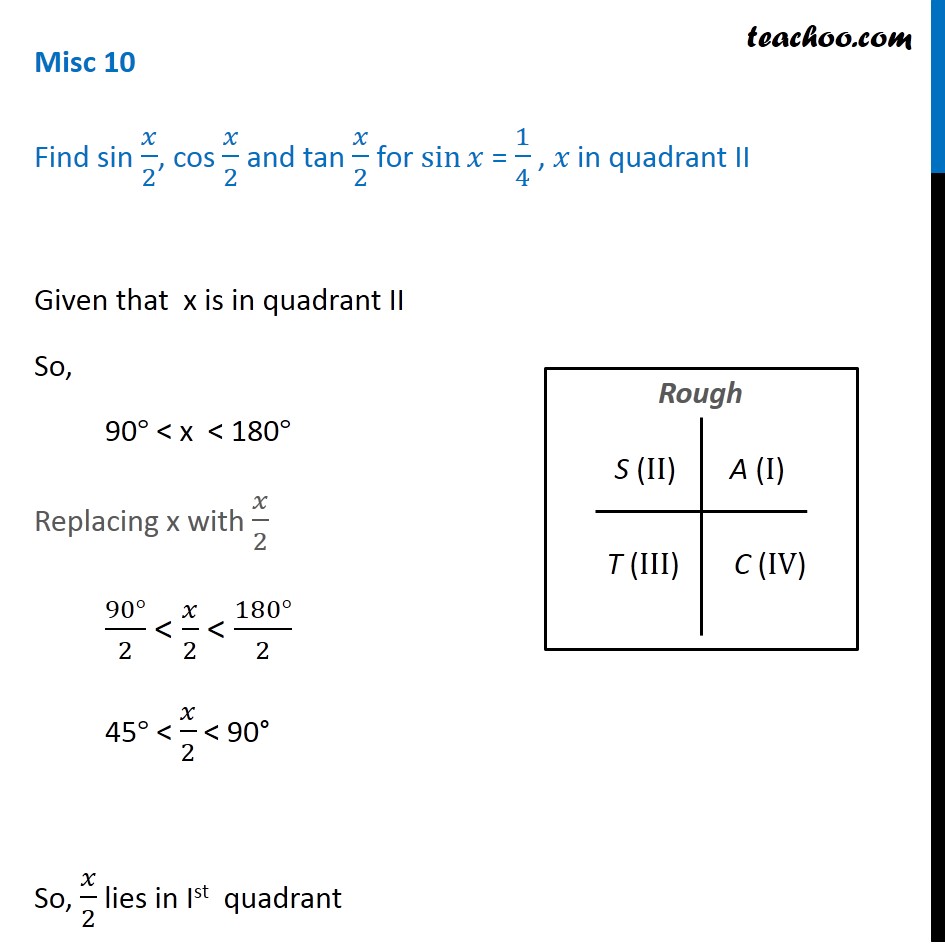



Misc 10 Sin X 1 4 Find Sin X 2 Cos X 2 Tan X 2 Chapter 3
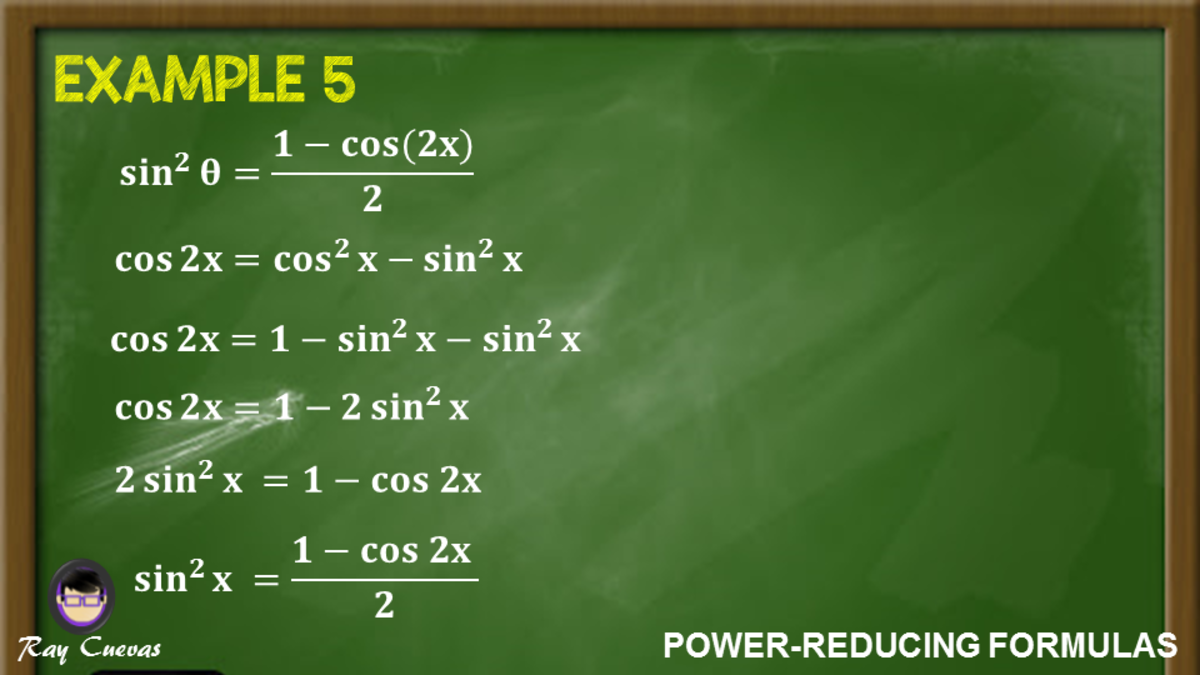



Power Reducing Formulas And How To Use Them With Examples Owlcation




Cos X Plus Sin X Upon Cos X Minus Sin X Minus Cos X Minus Sin X Upon Cos X Plus Sin X Is Equal To 2 Brainly In



Wl Apsva Us Wp Content Uploads Sites 38 15 05 Lesson 6 4 Notes 1 Pdf



Solved A First Rewrite The Left Hand Side 1 Tan 2x In Terms Of Sin X And Cos X B Next Add The Two Terms In The Expression You Got In Part



Www Richlandone Org Cms Lib Sc Centricity Domain 2228 Pc third quarter test review Pdf




How To Write Double Angle Formula Sin 2x In Terms Of Tan X Youtube



Http Www Tvschools Org Userfiles 281 Classes 9518 5 5 Pdf



Http Ww2 Justanswer Com Uploads Ryanp97 12 04 05 Solutions3 Pdf




Evaluate The Integral T Tan 2x Cos 3x Dx A Gauthmath




Sin 2 X Dawonaldson




Ppt Analytic Trig Powerpoint Presentation Free Download Id



Www Birmingham K12 Mi Us Cms Lib Mi Centricity Domain 976 Chapter 5 Pdf
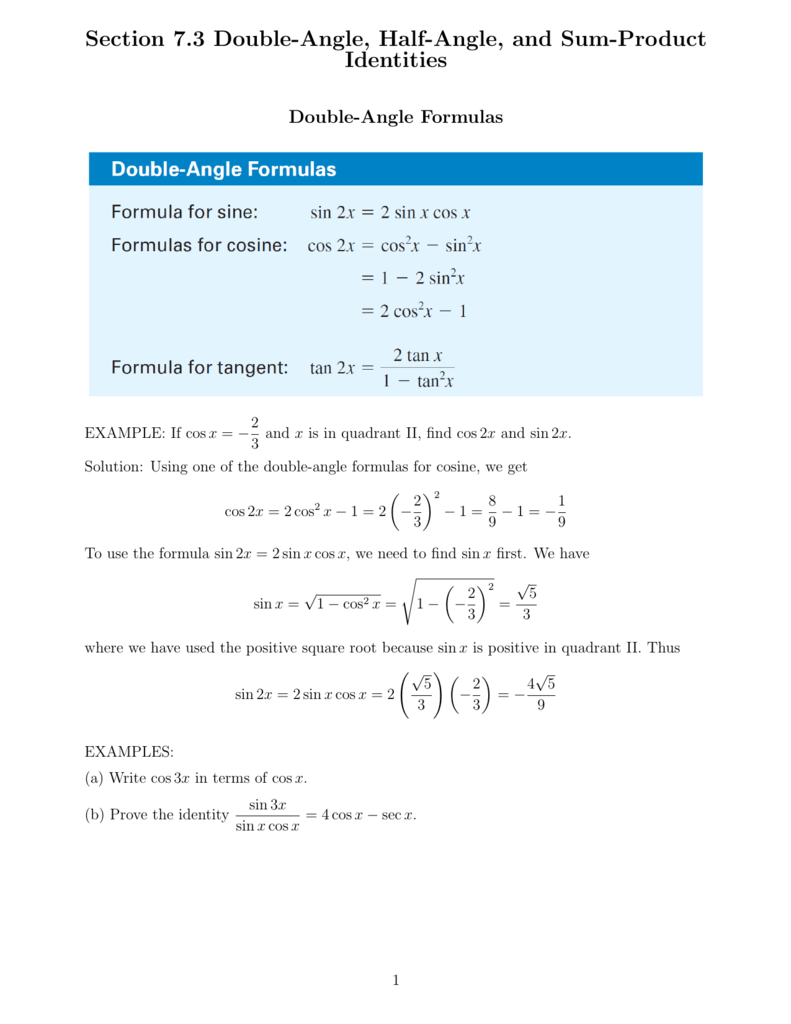



Section 7 3 Double Angle Half Angle And Sum




Verifying A Trigonometric Identity Cos X 1 Tan 2 X Cos X Sec X 1 Youtube



2




How Does One Verify Cos 2x Sin 2x 1 Tan 2x Cos 2x Socratic
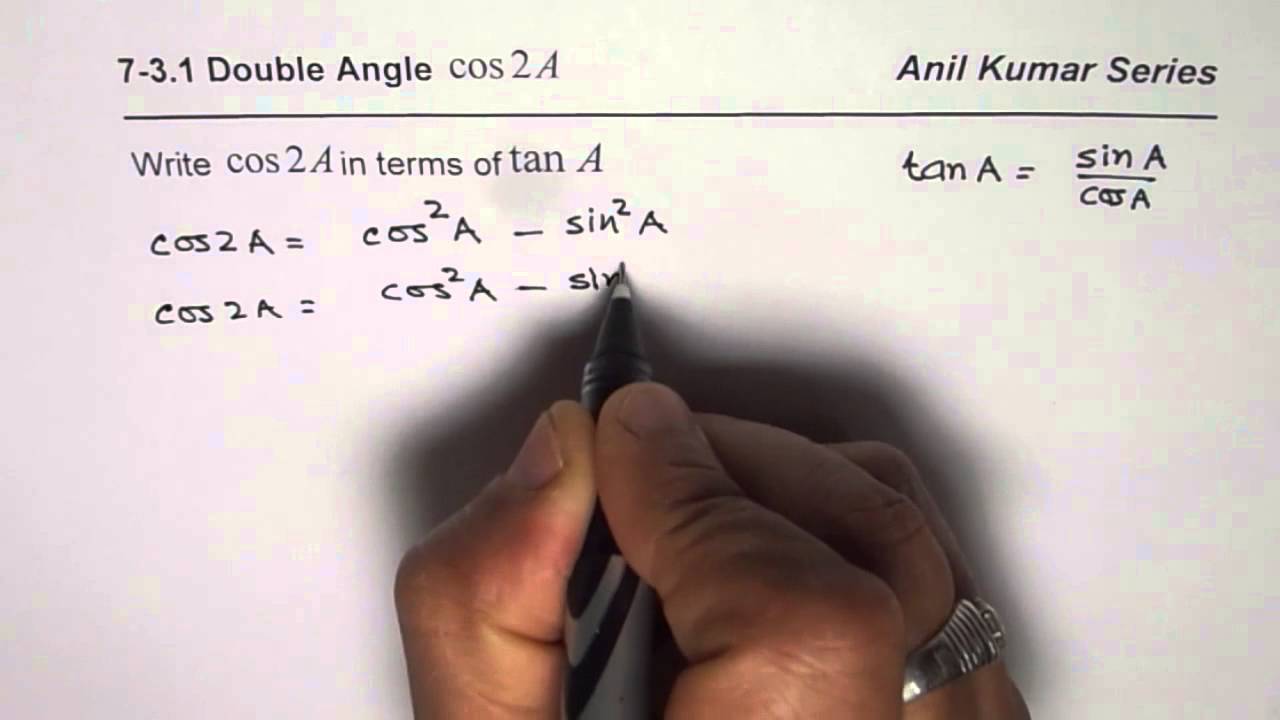



Write Cos2x In Terms Of Tanx Double Angle Transformation Youtube




Cosx 3 5 Find Tanx 2 Half Angle Tangent Formula Derivation With Important Example Youtube




Tan 2x Tan 2x



Http Www Pstcc Edu Facstaff Jwlamb 1730 7 3 Pdf
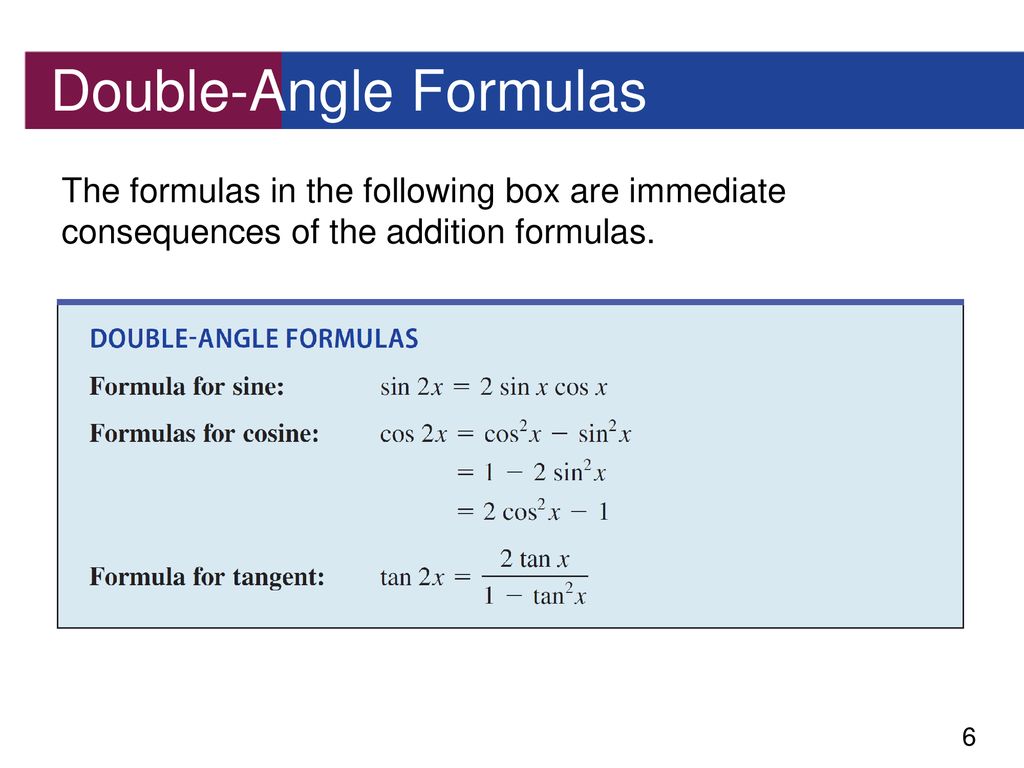



Copyright C Cengage Learning All Rights Reserved Ppt Download



Www Stewartcalculus Com Data Calculus concepts and contexts Upfiles 3c3 Trigonometintegrals Stu Pdf
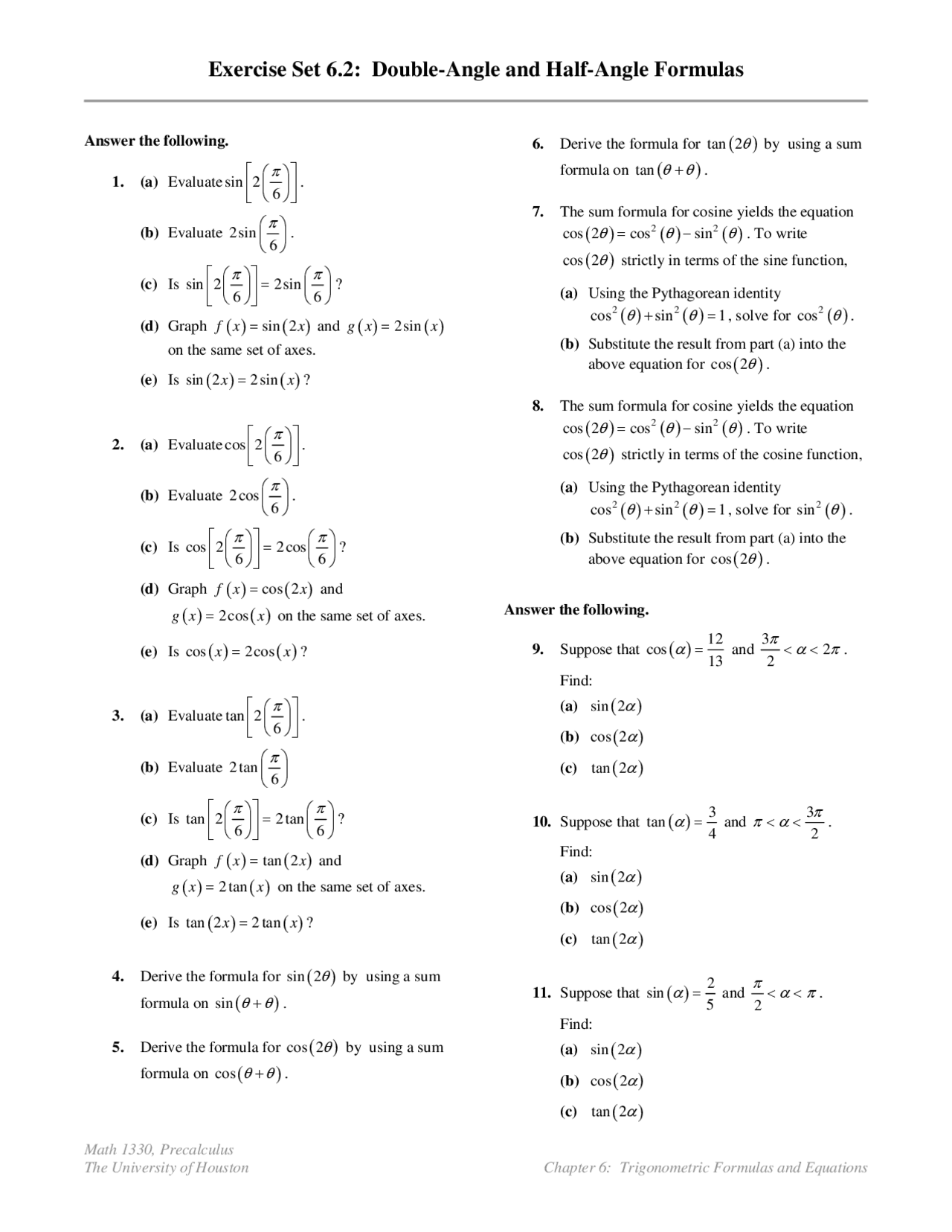



56 Questions On Double Angle And Half Angle Formula Precalculus Math 1330 Docsity



Http Pnhs Psd2 Org Documents Syoesle Pdf



Http Www Mayfieldschools Org Downloads 4 8 double angle formulas key Pdf



No comments:
Post a Comment